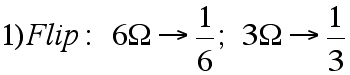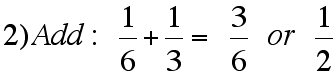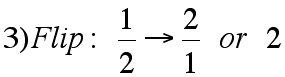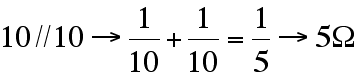|
|
Discussion: Equivalent resistance can be thought of as the question "How can I combine these two resistors into one resistor and not effect the behavior of the circuit?" There are two basic configurations for resistors: series and parallel. 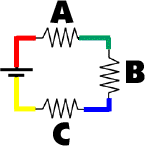 Resistors in series can simply be "added up." For example, the diagram to the left shows an original circuit with three resistors: A, B, and C. If you wanted to combine these, the equivalent resistor would simply be A+B+C ohms. Resistors in series can simply be "added up." For example, the diagram to the left shows an original circuit with three resistors: A, B, and C. If you wanted to combine these, the equivalent resistor would simply be A+B+C ohms.The colors are drawn in as part of "Mr. Bourne's Color Coding System™" For those curious, take a marker, start at the battery, and trace any wire you come across until you hit another component (like a resistor). If you have to cross a resistor, change colors. Series resistors suddenly become more obvious. A (Red-Green) is in series with B (Green-Blue) which is in series with C (Blue-Yellow). This system works well with simple circuits and helps you get the hang of it.
Again, let's take the parallel circuit in the diagram. Step 1: "Flip" the values. For example: "A" becomes "1/A" and "B" becomes "1/B." (Reciprocals) Step 2: "Add" the fractions. Step 3: "Flip" again! Here, let's try this numerically, combining two resistors, 3-ohm and 6-ohm:
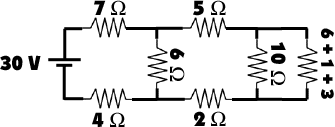
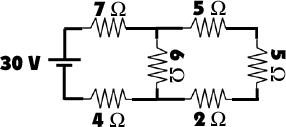
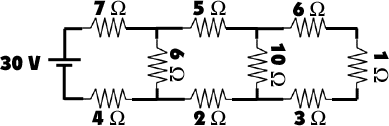
There! A 3-ohm and 6-ohm in parallel can be combined to form a 2-ohm. Now, let's go back to the original question. How can be combine this circuit. Hint: start at the right side, furthest away from the battery. Mentally color code if that helps too:
You should have seen the 6-ohm, 1-ohm, and 3-ohm on the right, and identified that they are in series. They can therefore be combined like so:
Now look at the circuit. The 10-ohm that you just "made" is in parallel with the other 10-ohm. Flip-Add-Flip!
Already, the circuit is looking simpler. Keep simplifying in this manner until you have only one resistor and the battery. You should have calculated an equivalent resistance of 15-ohms.
|
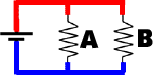 Resistors in parallel, such as those in the diagram to the right, can be combined with Mr. duBourg's "Flip-Add-Flip" trick. But before we get to that, notice Mr. Bourne's color coding here. Again, we started from the battery with a red marker, traced all of the wires, and changed color when we "crossed" something. Resistors A (Red-Blue) and B (Red-Blue) will be combined in parallel because they have the same color relations. Now onto combining in parallel:
Resistors in parallel, such as those in the diagram to the right, can be combined with Mr. duBourg's "Flip-Add-Flip" trick. But before we get to that, notice Mr. Bourne's color coding here. Again, we started from the battery with a red marker, traced all of the wires, and changed color when we "crossed" something. Resistors A (Red-Blue) and B (Red-Blue) will be combined in parallel because they have the same color relations. Now onto combining in parallel: